IAS Program on Inverse Problems, Imaging and Partial Differential Equations

The Calderón Problem for Quasilinear Conductivities

Prof. Yavar KIAN, Associate Professor, Centre de Physique Théorique, Aix-Marseille University

Applied Mathematics Computer Engineering Inverse Problems Partial Differential Equations

Abstract

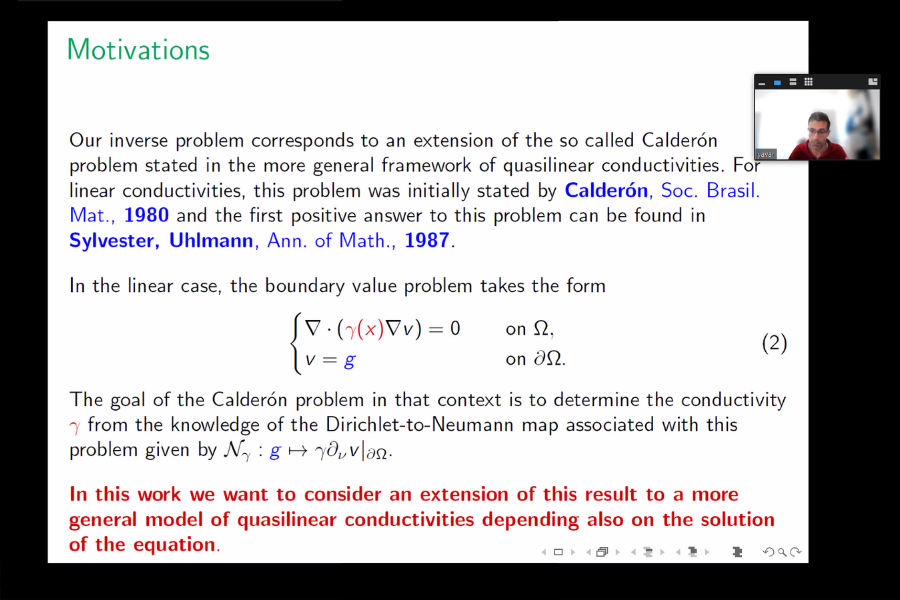
In this talk, the speaker will consider the Calderón inverse problem of determining uniquely a quasilinear isotropic conductivity appearing in an elliptic equation on a bounded open set. More precisely, they consider the problem of determining a general quasi-linear conductivity depending simultaneously on the space variable, the solutions and the gradient of the solutions of an elliptic non-linear equation. The speaker will give a positive answer to this problem for some general class of conductivities subjected to some analytic dependency with respect to the gradient of the solutions of the equation. This talk is based on a joint work with Catalin CARSTEA, Ali FEIZMOHAMMADI, Katya KRUPCHYK and Gunther UHLMANN.

For Attendees' Attention

This talk is hosted by the Department of Mathematics of the Chinese University of Hong Kong and will be held online via Zoom. To attend, please join the Zoom meeting at https://cuhk.zoom.us/j/98241093146 (Meeting ID: 982 4109 3146).

About the Program

For more information, please refer to the program website at https://iasprogram.hkust.edu.hk/inverseproblems/.

The Calderón Problem for Quasilinear Conductivities

Event Information