Radiative Transfer Equation for Surface and Body Waves
Abstract
The motivation of this talk is to study the propagation of seismic waves in Earth's crust and to derive a radiative transfer equation that accounts for body and surface waves.
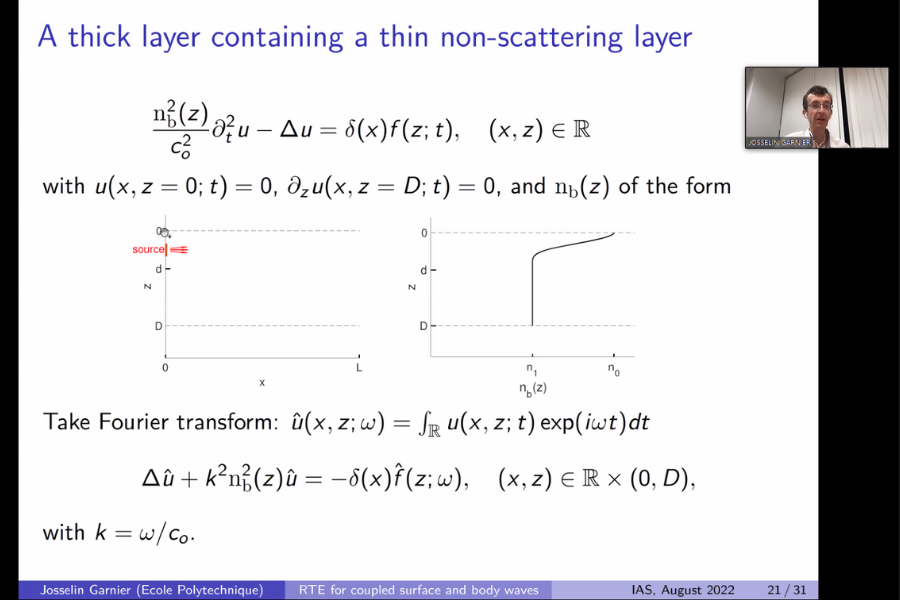
The model considered in this talk is an acoustic wave equation in a randomly heterogeneous two-dimensional half-space with reflecting boundary conditions and with a thin layer near the surface. There are two types of modes that can propagate: those that are almost trapped in the thin layer, and thus model surface waves, and those that penetrate deep into the medium, and thus model body waves. The speaker and his research group will show how the wave becomes incoherent (its mean goes to zero) while the incoherent wave fluctuations can be described by a radiative transfer equation satisfied by the mean Wigner transform of the wave field. They will describe some properties of the radiative transfer equation including a nontrivial coupling mechanism between body modes mediated by surface modes and the existence of a slowly evolving metastable surface mode distribution which ultimately leads to energy equipartition between all modes.
This is based on joint work with L. BORCEA (University of Michigan), M. V. DE HOOP (Rice University), and K. SOLNA (University of California, Irvine).
About the Speaker
Prof. Josselin GARNIER received his PhD from the École Polytechnique in 1996 and continued his research there as a CNRS Research Fellow until 2001. He joined the Université Toulouse III - Paul Sabatier as an Associate Professor in 2001 and then the Université Paris Diderot in 2005, where he was promoted to Professor in 2007. In 2016, he returned to the École Polytechnique and is currently a Professor in Applied Mathematics. He is also the Vice Chair of the Department of Applied Mathematics there.
Prof. Garnier’s research interests concern various aspects of applied probability and stochastic modeling, including wave propagation in random media, imaging for waves in complex media, stochastic and evolutionary algorithms, uncertainty quantification, and risk analysis. He is the editor of the book series, Modelling and Simulation in Medical Imaging, and a member of the editorial boards of journals including Asymptotic Analysis, Discrete and Continuous Dynamical Systems - Series S, ESAIM: Probability and Statistics, Forum Mathematicum, SIAM Journal on Applied Mathematics, and SIAM/ASA Journal on Uncertainty Quantification.
Prof. Garnier is a recipient of the Blaise Pascal Prize of the French Academy of Sciences (2007) and the Felix Klein Prize of the European Mathematical Society (2008). In 2021, he was awarded the Grand Prix Scientique by the Simone and Cino Del Duca Foundation.
For Attendees' Attention
This talk will be held online via Zoom. To attend, please join the Zoom meeting at https://hkust.zoom.us/j/92884946324 (Meeting ID: 928 8494 6324 / Passcode: iasip2022).
About the Program
For more information, please refer to the program website at https://iasprogram.hkust.edu.hk/inverseproblems/.