An Inverse Source Problem for Electromagnetic Waves

Abstract

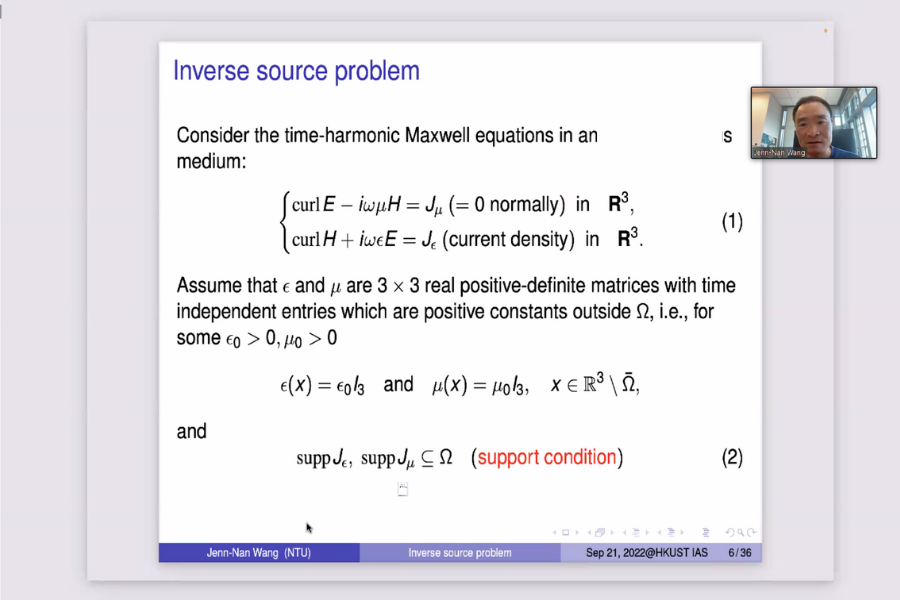
In this talk, the speaker would like to discuss the uniqueness and the increasing stability in the inverse source problem for electromagnetic waves in homogeneous and inhomogeneous media from boundary data at multiple wave numbers. For the unique determination of sources, he and his research group consider inhomogeneous media and use tangential components of the electric field and magnetic field at the boundary of the reference domain. The proof relies on the Fourier transform with respect to the wave numbers and the unique continuation theorems. To study the increasing stability in the source identification, they consider homogeneous media and measure the absorbing data or the tangential component of the electric field at the boundary of the reference domain as additional data. By using the Fourier transform with respect to the wave numbers, explicit bounds for analytic continuation, Huygens' principle and bounds for initial boundary value problems, increasing (with larger wave numbers intervals) stability estimate is obtained. This is a joint work with Victor ISAKOV.

About the Speaker

Prof. WANG Jenn-nan received his PhD in Mathematics from the University of Washington in 1997. He then returned to Taiwan and continued his research as a Postdoctoral Fellow in the National Chung Cheng University. In 1998, he joined the National Cheng Kung University as an Assistant Professor and then moved to the National Taiwan University (NTU) as an Associate Professor in 2002. He is currently a Distinguished Professor in the Institute of Applied Mathematical Sciences at the Department of Mathematics in NTU.

Prof. Wang’s research interest is in partial differential equations and inverse problems. He is the Editor-in-Chief of Taiwanese Journal of Mathematic and the Associate Editor of the Journal of Mathematical Analysis and Applications.

For Attendees' Attention

This talk will be held online via Zoom. To attend, please join the Zoom meeting at https://hkust.zoom.us/j/91205115224 (Meeting ID: 912 0511 5224 / Passcode: iasip2022).

About the Program

For more information, please refer to the program website at https://iasprogram.hkust.edu.hk/inverseproblems/.